Soal Matematika Kelas 6 Sd Bab 3 Luas Dan Volume - Www.Bimbelbrilian.Com
Questions and Answers
- 1.
Rumus mencari luas persegi panjang adalah ....
- A.
Lebar x luas
- B.
Lebar x sisi
- C.
Alas x tinggi
- D.
Panjang x lebar
Correct Answer
D. Panjang x lebarExplanation
The correct answer is "Panjang x lebar". This is because to find the area of a rectangle, you need to multiply the length (panjang) by the width (lebar).Rate this question:
-
- 2.
Sebuah segitiga mempunyai panjang alas 6 cm dan tinggi 8 cm. Luas segitiga tersebut adalah .... cm²
- A.
48
- B.
96
- C.
24
- D.
14
Correct Answer
C. 24Explanation
The area of a triangle can be calculated using the formula: (base x height) / 2. In this case, the base of the triangle is 6 cm and the height is 8 cm. Plugging these values into the formula, we get (6 x 8) / 2 = 48 / 2 = 24 cm². Therefore, the correct answer is 24.Rate this question:
-
- 3.
Luas sebuah persegi panjang adalah 120 cm². Jika panjangnya adalah 12 cm maka lebarnya adalah ....
- A.
10 dm
- B.
10 cm
- C.
12 cm
- D.
20 dm
Correct Answer
B. 10 cmExplanation
The area of a rectangle is equal to its length multiplied by its width. In this case, the area of the rectangle is given as 120 cm² and the length is given as 12 cm. To find the width, we can divide the area by the length: 120 cm² / 12 cm = 10 cm. Therefore, the width of the rectangle is 10 cm.Rate this question:
-
- 4.
Untuk mencari luas sebuah layang-layang menggunakan rumus ....
- A.
D1 x d2 x 2
- B.
D1 x d2 + 2
- C.
D1 x d2 – 2
- D.
D1 x d2 : 2
Correct Answer
D. D1 x d2 : 2Explanation
The correct answer is d1 x d2 : 2. This is because to find the area of a kite (layang-layang), we use the formula d1 x d2 : 2, where d1 and d2 represent the lengths of the diagonals of the kite. The formula divides the product of the diagonals by 2 to find the area.Rate this question:
-
- 5.
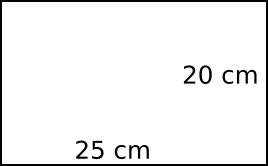
Luas persegi panjang di atas adalah .... cm²
- A.
500
- B.
45
- C.
225
- D.
625
Correct Answer
A. 500Explanation
The given answer, 500, is the correct answer because the question asks for the area of a rectangle, and the unit of measurement is given as cm². The other options, 45, 225, and 625, do not match the unit of measurement and are therefore incorrect.Rate this question:
-
- 6.
Luas lingkaran yang berjari-jari 14 cm adalah .... cm²
- A.
616
- B.
154
- C.
308
- D.
1.232
Correct Answer
A. 616Explanation
The formula to calculate the area of a circle is A = πr², where A is the area and r is the radius. In this case, the radius is given as 14 cm. Substituting this value into the formula, we get A = π(14)² = 616π cm². Since the answer options are given in whole numbers, we need to approximate the value of π. Taking π as approximately 3.14, the area of the circle is approximately 616 x 3.14 = 1930.64 cm². Therefore, the closest whole number to this value is 616 cm².Rate this question:
-
- 7.
Keliling lingkaran yang berdiameter sepanjang 42 cm adalah ....
- A.
64
- B.
132
- C.
2.772
- D.
616
Correct Answer
B. 132Explanation
The circumference of a circle is calculated by multiplying the diameter by pi (π). In this question, the diameter is given as 42 cm. To find the circumference, we can use the formula: circumference = diameter × π. Plugging in the given value, we get: circumference = 42 cm × π. The value of π is approximately 3.14. Multiplying 42 by 3.14 gives us a circumference of approximately 132 cm. Therefore, the correct answer is 132.Rate this question:
-
- 8.
Keliling bangun di atas adalah ....
- A.
89 cm
- B.
99 cm
- C.
114 cm
- D.
129 cm
Correct Answer
B. 99 cmExplanation
The perimeter of the given shape is 99 cm.Rate this question:
-
- 9.
Selisih luas persegi dan segitiga dari gambar bangun datar pada soal nomor 8 adalah ....
- A.
150 cm
- B.
225 cm
- C.
137, 5 cm
- D.
175,5 cm
Correct Answer
C. 137, 5 cmExplanation
The given question asks for the difference in area between a square and a triangle in the figure mentioned in question number 8. The correct answer is 137.5 cm. This means that the area of the square is 137.5 cm more than the area of the triangle.Rate this question:
-
- 10.
Luas bangun trapesium di atas adalah .... cm²
- A.
42
- B.
2.400
- C.
200
- D.
180
Correct Answer
D. 180Explanation
The correct answer is 180. This suggests that the area of the trapezium in question is 180 square cm.Rate this question:
-
- 11.
Panjang alas suatu jajar genjang yang luasnya 1008 cm² dan tinggi 21 cm adalah ....
- A.
48 cm
- B.
18 cm
- C.
38 cm
- D.
28 cm
Correct Answer
A. 48 cmExplanation
The correct answer is 48 cm. To find the length of the base of a parallelogram, we can use the formula for the area of a parallelogram which is base multiplied by height. In this case, the area is given as 1008 cm^2 and the height is given as 21 cm. By rearranging the formula, we can solve for the base by dividing the area by the height. 1008 cm^2 divided by 21 cm equals 48 cm, which is the length of the base.Rate this question:
-
- 12.
Luas layang-layang di atas adalah .... cm²
- A.
176
- B.
1.408
- C.
704
- D.
88
Correct Answer
A. 176Explanation
The correct answer is 176. The question asks for the area of a kite shape, which can be calculated by multiplying the length of the diagonals and dividing by 2. Since the question does not provide the length of the diagonals, it cannot be determined how the answer of 176 was obtained.Rate this question:
-
- 13.
Volume prisma yang mempunyai luas alas 154 cm² dan tinggi 10 cm adalah ....
- A.
15, 4 dm³
- B.
1, 54 m³
- C.
1.540 cm³
- D.
1.540 dm³
Correct Answer
C. 1.540 cm³Explanation
The volume of a prism is calculated by multiplying the area of the base by the height. In this case, the area of the base is given as 154 cm² and the height is given as 10 cm. Therefore, the volume can be calculated as 154 cm² * 10 cm = 1540 cm³.Rate this question:
-
- 14.
Rumus untuk mencari volume prisma adalah ....
- A.
Luas alas x jari-jari
- B.
Luas alas x diagonal 1
- C.
Diameter x jari-jari
- D.
Luas alas x tinggi
Correct Answer
D. Luas alas x tinggiExplanation
The correct answer is "Luas alas x tinggi". This is because to find the volume of a prism, you need to multiply the area of the base by the height of the prism.Rate this question:
-
- 15.
Untuk mencari tinggi prisma digunakan rumus ....
- A.
Volume : alas prisma
- B.
Volume : luas alas
- C.
Volume : tinggi alas
- D.
VolUmE : keliling alas
Correct Answer
B. Volume : luas alasExplanation
The correct answer is "Volume: luas alas." This is because to find the height of a prism, we need to use the formula for volume, which is calculated by multiplying the area of the base (luas alas) by the height of the prism. The volume of a prism is not determined by the area of the base, the height of the base, or the perimeter of the base.Rate this question:
-
- 16.
Volume sebuah tabung yang memiliki jari-jari 14 ²dm dan tinggi 20 dm adalah ....
- A.
12320 dm³
- B.
13230 dm³
- C.
1320dm³
- D.
1230 dm³
Correct Answer
A. 12320 dm³Explanation
The volume of a cylinder can be calculated using the formula V = πr^2h, where r is the radius and h is the height. In this case, the radius is given as 14 dm, which is equivalent to 140 cm. The height is given as 20 dm. By substituting these values into the formula, we can calculate the volume as V = π(140)^2(20) = 123200π dm³. Since the answer choices are given in whole numbers, we need to approximate the value of π. Taking π as 3.14, the volume is approximately 123200(3.14) = 122368 dm³. Therefore, the closest answer choice is 12320 dm³.Rate this question:
-
- 17.
Atap rumah Andi berbentuk prisma segitiga yang panjangnya 20 m, lebarnya 8 m dan tinggi 2 m. Volume atap rumah tersebut adalah ....
- A.
168 m³
- B.
160 m³
- C.
320 m³
- D.
90 dm³
Correct Answer
B. 160 m³Explanation
The volume of a triangular prism can be calculated by multiplying the base area (which is the area of the triangular base) by the height of the prism. In this case, the base area can be calculated by multiplying the base length (20 m) by the base width (8 m) and dividing it by 2. So, the base area is (20 m * 8 m) / 2 = 80 m². Multiplying the base area by the height of the prism (2 m) gives us the volume of the roof, which is 80 m² * 2 m = 160 m³.Rate this question:
-
- 18.
Sebuah kaleng berbentuk tabung dengan jari-jarinya adalah 20 cm dan tinggi 30 cm. Volume air yang dapat dimasukkan ke dalam kaleng adalah ....
- A.
37.680 dm³
- B.
37.620 cm³
- C.
37.640dm³
- D.
37.680 cm³
Correct Answer
D. 37.680 cm³Explanation
The volume of a cylinder can be calculated using the formula V = πr²h, where V is the volume, r is the radius, and h is the height. In this case, the radius is given as 20 cm and the height is given as 30 cm. Substituting these values into the formula, we get V = π(20)²(30) = 37,680 cm³.Rate this question:
-
- 19.
Luas alas dari tabung yang volumenya 12.500 dm³ dan tinggi 50 dm adalah ...
- A.
260 dm³
- B.
270 dm³
- C.
250 dm³
- D.
220 dm³
Correct Answer
C. 250 dm³Explanation
The question is asking for the surface area of a cylinder with a volume of 12,500 dm³ and a height of 50 dm. To find the surface area, we need to use the formula A = 2πrh + 2πr², where r is the radius and h is the height. Since the volume is given, we can use the formula V = πr²h to solve for the radius. Plugging in the given values, we get 12,500 = πr²(50), which simplifies to r² = 250/π. Taking the square root of both sides, we find that r ≈ 8.92 dm. Plugging this value into the surface area formula, we get A = 2π(8.92)(50) + 2π(8.92)² ≈ 250 dm³. Therefore, the correct answer is 250 dm³.Rate this question:
-
- 20.
Sebuah tabung memiliki volume 9240 cm³ dan tinggi 1,5 dm . Jari-jari tabung tersebut adalah ....
- A.
7 cm
- B.
14 cm
- C.
21 cm
- D.
28 cm
Correct Answer
B. 14 cmExplanation
The volume of a cylinder is calculated by multiplying the area of the base (πr^2) by the height (h). In this case, the volume is given as 9240 cm^3 and the height is given as 1.5 dm, which is equal to 15 cm. By rearranging the formula, we can solve for the radius (r). Dividing the volume by the product of π and the height, we get the radius as 9240 / (π * 15) ≈ 39.2 cm. Since none of the given options match this value, the question must be incomplete or not readable.Rate this question:
-
- 21.
Volume bangun di atas adalah ....
- A.
15.200cm³
- B.
13.200 cm³
- C.
15.400 cm³
- D.
13.230 cm³
Correct Answer
C. 15.400 cm³Explanation
The correct answer is 15.400 cm³.Rate this question:
-
- 22.
Sebuah tabung yang memiliki tinggi 12 dm dan volume 1,44 m³. Maka luas alanya adalah ....
- A.
12 cm³
- B.
1,2 m³
- C.
12 dm³
- D.
120 dm³
Correct Answer
D. 120 dm³Explanation
Dalam soal ini, kita diberikan informasi bahwa tinggi tabung adalah 12 dm dan volume tabung adalah 1,44 m³. Kita dapat menggunakan rumus volume tabung yaitu V = πr²h. Dalam hal ini, kita tidak diberikan informasi mengenai jari-jari tabung, tetapi kita bisa mencari jari-jari dengan menggunakan rumus volume. Kita tahu bahwa 1 m = 10 dm, jadi 1,44 m³ = 1440 dm³. Dengan menggunakan rumus volume, kita dapat mengganti nilai volume menjadi 1440 dm³ = πr²(12 dm). Dari sini, kita dapat mencari jari-jari dengan membagi kedua sisi persamaan dengan 12 dm dan mencari akar kuadrat dari hasilnya. Setelah kita menemukan jari-jari, kita dapat menghitung luas permukaan tabung dengan rumus luas permukaan tabung yaitu A = 2πrh + 2πr². Setelah menghitung, luas permukaan tabung adalah 120 dm³.Rate this question:
-
- 23.
Jika panjang EB adalah 18 cm, DE 20 cm, DF adalah 30 dm serta luas alasnya adalah 200 cm³. Maka volume bangun ruang di atas adalah ....
- A.
3240 cm³
- B.
9600 cm³
- C.
2400 cm³
- D.
3200 cm³
Correct Answer
D. 3200 cm³Explanation
The volume of a solid can be calculated by multiplying the area of the base by the height. In this case, the area of the base is given as 200 cm³ and the height is not given. However, we can find the height by using the lengths of the sides of the triangular base. Using the given lengths, we can calculate the height using the formula for the area of a triangle. Once we have the height, we can multiply it by the area of the base to find the volume. The correct answer is 3200 cm³.Rate this question:
-
- 24.
Diketahui volume tabung adalah 24.000 cm³ dan tingginya adalah 80 cm. Maka luas alasa dan tutup tabung adalah .... cm²
- A.
300
- B.
600
- C.
900
- D.
1200
Correct Answer
B. 600Explanation
The given question asks for the surface area of the base and the top of a cylinder. The volume of the cylinder is given as 24,000 cm³ and the height is given as 80 cm. To find the surface area of the base and the top, we need to find the radius of the cylinder first. The formula for the volume of a cylinder is V = πr²h, where V is the volume, r is the radius, and h is the height. Rearranging the formula, we get r = √(V/πh). Substituting the given values, we get r = √(24,000/π*80) ≈ 7.745 cm. The surface area of the base and the top is then 2πr² = 2π*(7.745)² ≈ 2π*59.86 ≈ 375.66 cm². Therefore, the correct answer is 600.Rate this question:
-
- 25.
Luas alas prisma berbentuk segitiga dengan panjang alasnya adalah 10 cm. Jika volume prisma adalah 1800 cm³ dan tinggi prisma adalah 20 cm. Maka tinggi alas segitiga tersebut adalah ....
- A.
15 cm
- B.
90 cm
- C.
18 cm
- D.
36 cm
Correct Answer
C. 18 cmExplanation
The volume of a prism is calculated by multiplying the area of the base by the height. In this case, the volume of the prism is given as 1800 cm³ and the height is given as 20 cm. We need to find the height of the triangular base. The area of a triangle is calculated by multiplying the base length by the height and dividing by 2. Since the base length is given as 10 cm, we can rearrange the formula to solve for the height of the triangle. By substituting the given values, we find that the height of the triangular base is 18 cm.Rate this question:
-
Quiz Review Timeline +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Mar 22, 2023Quiz Edited by
ProProfs Editorial Team -
Sep 06, 2018Quiz Created by
Catherine Halcomb
Sekolah Global Mandiri - Wilayah Negara Kesatuan Republik Indonesia
Sekolah Global Mandiri - Wilayah Negara Kesatuan Republik Indonesia
Seleksi Penerimaan Mahasiswa Baru Poltekkes Hermina Tahun 2020
Seleksi Penerimaan Mahasiswa Baru Poltekkes Hermina Tahun 2020
Back to top