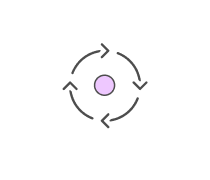
Angular velocity is expressed in units of
Angular acceleration is expressed in units of
Two equal forces are applied to a door at the doorknob. The first...
A wheel of radius 1.0 m is rotating with a constant angular speed of...
Angular displacement is usually express in units of
A 0.300-kg mass, attached to the end of a 0.750-m string, is whirled...
A wheel of diameter of 68.0 cm slows down uniformly from 8.40 m/s to...
A wheel with moment of inertia 3.00 kg*m^2 has a net torque of 3.50...
A bicycle wheel rotates uniformly through 2.0 revolutions in 4.0 s....
The bolts on a car wheel require tightening to a torque of 90 N*m. If...
Consider a motorcycle of mass 150 kg, one wheel of which has a mass of...
The second hand of a clock has a length of 0.30 m. What distance does...
A uniform solid sphere has mass M and radius R. If these are increased...
How many rad/s is 25 revolutions per minute equivalent to?
An ice skater performs a pirouette (a fast spin) by pulling in his...
An object's angular momentum changes by 20 kg*2/s in 4.0 s. What...
A bicycle wheel rotates uniformly through 2.0 revolutions in 4.0 s....
A bicycle wheel rotates uniformly through 2.0 revolutions in 4.0 s....
What is the quantity used to measure an object's resistance to...
The Earth moves about the Sun in an elliptical orbit. As the Earth...
An ice skater has a moment of inertia of 5.0 kg*m^2 when her arms are...
A wheel of diameter 26 cm turns at 1500 rpm. How far will a point on...
A car is negotiating a flat circular curve of radius 50 m with a speed...
A wheel of diameter of 68.0 cm slows down uniformly from 8.40 m/s to...
The bolts on a car wheel require tightening to a torque of 90 N*m. If...
A boy and a girl are riding on a merry-go-round which is turning at a...
A wheel accelerates with a constant angular acceleration of 4.5...
A boy and a girl are riding on a merry-go-round which is turning at a...
Two equal forces are applied to a door. The first force is applied at...
Consider a bicycle wheel to be a ring of radius 30 cm and mass 1.5 kg....
The cutting cord on a gas-powered weed cutter is 0.16 m in length. If...
Consider two uniform solid spheres where both have the same diameter,...
If a constant net torque is applied to an object, that object will
The Earth orbits the Sun in an elliptical orbit. Ignore any friction...
A wheel of moment of inertia of 5.00 kg*m^2 starts from rest and...
A solid sphere of mass 1.0 kg and radius 0.010 m rolls with a speed of...
A figure skater rotating at 5.00 rad/s with arms extended has a moment...
A car is negotiating a flat circular curve of radius 50 m with a speed...
A wheel of diameter of 68.0 cm slows down uniformly from 8.40 m/s to...
The moment of inertia of a solid cylinder about its axis is given by...
A boy and a girl are riding a merry-go-round which is turning at a...
Consider two uniform solid spheres where one has twice the mass and...
A 1.53-kg mass hangs on a rope wrapped around a frictionless disk...
A cable car at a ski resort carries skiers a distance of 6.8 km. The...
Rolling without slipping depends on
How many revolutions per minute (rpm) must a circular, rotating space...
Two forces are applied to a doorknob, perpendicular to the door. The...
A 4.00-m long rod is hinged at one end. The rod is initially held in...
What arc length does the Earth travel in a three month period in its...
A phonograph record rotates at 45 rpm. Through what angle does it turn...
A Ferris wheel rotating at 20 rad/s decelerates with a constant...
Consider a rigid body that is rotating. Which of the following is an...
Two uniform solid spheres have the same mass, but one has twice the...
A 1.53-kg mass hangs on a rope wrapped around a frictionless disk...
A wheel of radius 1.0 m is rotating with a constant angular speed of...
A triatomic molecule is modeled as follows: mass m is at the origin,...
A rotating flywheel can be used to store energy. If it is required to...
Suppose a solid sphere of mass M and radius R rolls without...
An ice skater performs a pirouette (a fast spin) by pulling in his...
A hoop of radius 0.50 m and a mass of 0.20 kg is released from rest...
A wheel starts at rest, and has an angular acceleration of 4 rad/s2....
A wheel starts from rest and reaches an angular speed of 6.0 rad/s...
A proton of mass 1.67 * 10^(-27) kg rotates with an angular speed...
"The total angular momentum of a system of particles changes when...
A boy and a girl are riding on a merry-go-round which is turning at a...
An ice skater performs a pirouette (a fast spin) by pulling in his...
A solid cylinder of mass 10 kg is pivoted about a frictionless axis...
In what circumstances can the angular velocity of system of particles...
A pulsar (a rotating neutron star) emits pulses at a frequency of 0.40...
What is the centripetal acceleration of a point on the perimeter of a...
A boy and a girl are riding a merry-go-round which is turning at a...
Suppose a solid sphere of mass M and radius R rolls without slipping...
A solid sphere of mass 1.0 kg and radius 0.010 m starts from rest and...
A wheel accelerates with a constant angular acceleration of 4.5...










 Back to top
Back to top